1. Trig Inversesap Calculus Problems

Derivatives of the Trig Functions. Implicit Differentiation. Inverse Trig Derivatives. Fundamental Theorem of Calculus, Part 1. Trapezoidal Rule. Vibration and Shock Handbook Mechanical Engineering Series Frank Kreith - Series Editor Published Titles Distributed Generation: The Power Paradigm for the New Millennium Anne-Marie Borbely & Jan F. Kreider Elastoplasticity Theor y Vlado A. Lubarda Energy Audit of Building Systems: An Engineering Approach Moncef Krarti Engineering Experimentation Euan Somerscales Entropy Generation.

Inverse Function Review

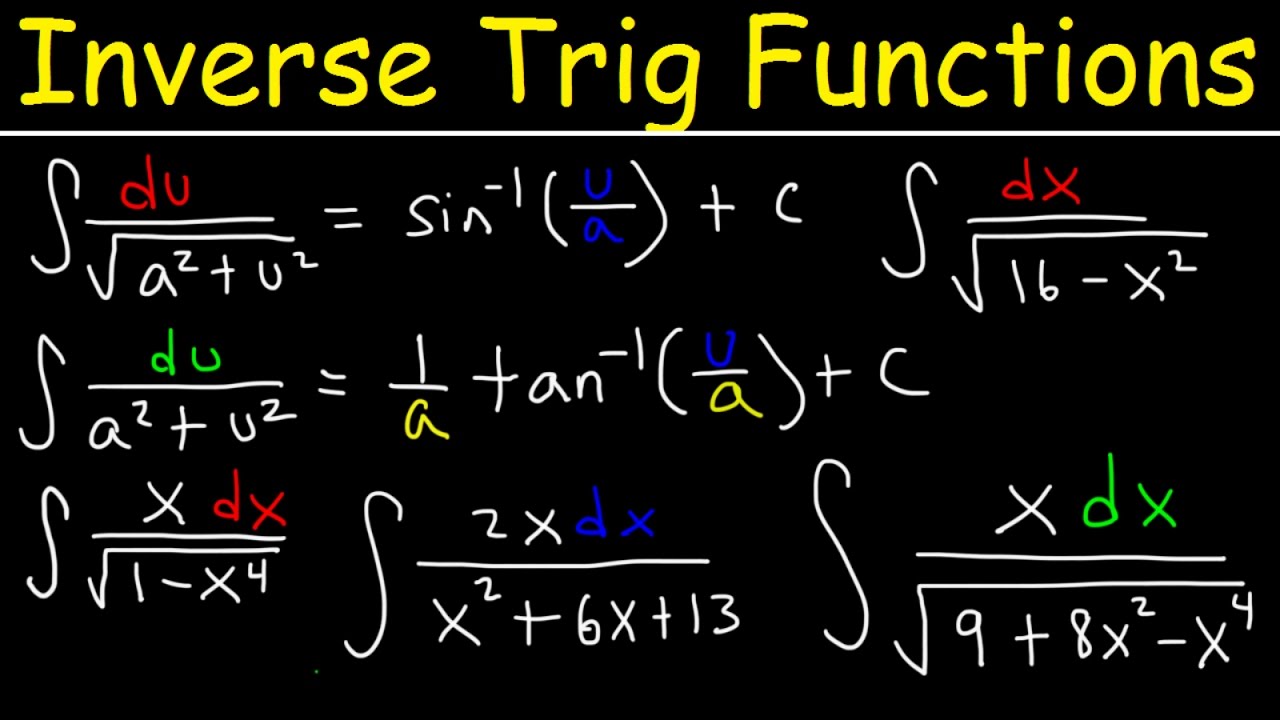
One application of the chain rule is to compute the derivative of an inverse function. First, let's review the definition of an inverse function:

We say that the function is invertible on an interval [a, b] if there are no pairsin the interval such thatand . That means there are no two x-values that have the same y-value. That's important, because if two x-coordinates map to the same y-coordinate, the inverse function (working in reverse) would map a single x-coordinate to multiple y-coordinates. That doesn't make sense, because f(x) could have more than one resulting value!

We say thatis the inverse of an invertible functionon [a, b] if:

For example, the functionsandare inverses onsinceon that interval. Note that it works both ways -- the inverse function of the original function returns x, and the original function performed on the inverse ALSO returns x.

Taking The Derivative

So, how do we differentiate an inverse function? Recall the chain rule:

Applying this to the definition of an inverse function, we have:

So:

Let's see how to apply this to real examples.

Example 1

Letsoas above. Then, and applying the formula we have:

This agrees with the answer we would get from viewingas the polynomial function.

Example 2

The functionis invertible on the interval, with inverse. We know that, so applying our formula we see that

We can check that , which means that

Trig Inversesap Calculus Problems

.